4x ^ 2 – 5x – 12 = 0 – Explore The Path To Solve Quadratic Equation

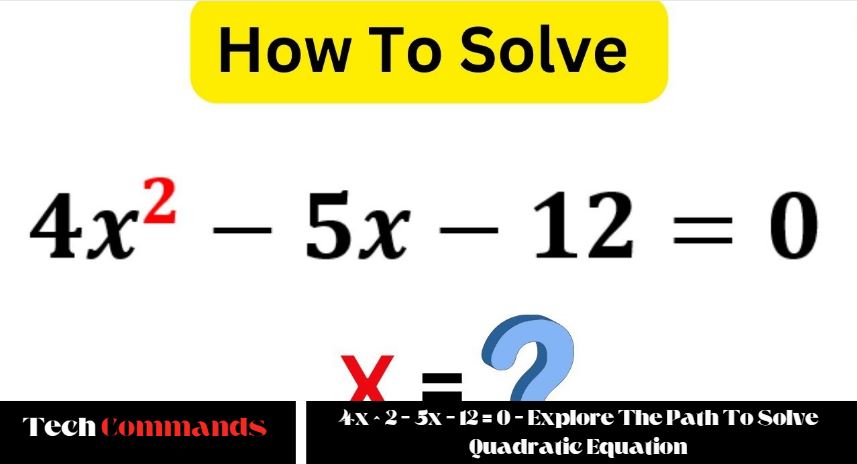
The quadratic equation 4x^2 – 5x – 12 = 0 represents a fundamental concept in algebra and mathematics, offering a gateway to understanding the behaviour of various functions and their solutions. Quadratic equations appear extensively in real-world scenarios, ranging from physics and engineering to economics and computer science. This particular equation presents a quadratic polynomial in standard form, where the highest power of the variable, x, is squared.

To solve this equation, one typically employs various methods, each providing insights into the nature of the solutions. One commonly used approach is the quadratic formula, which provides a direct method for finding the roots of any quadratic equation. Alternatively, one may explore factoring techniques, completing the square, or graphing methods to visualize the solutions.

The journey to solve this quadratic equation involves unravelling its complexities step by step. Whether through algebraic manipulations, graphical analysis, or numerical methods, each pathway offers a unique perspective on the problem. Moreover, understanding the properties of quadratic functions and their solutions lays the groundwork for tackling more advanced mathematical concepts.

What is this equation, and why is it important to solve?

The seemingly cryptic arrangement of numbers and variables in the equation “4x^2 – 5x – 12 = 0” belies its significance as a quadratic equation—a puzzle with far-reaching applications across various disciplines. Quadratic equations like this one serve as potent tools in deciphering real-world phenomena, from modelling projectile motion to charting celestial trajectories. They are the cryptographic keys that unveil solutions to practical problems, akin to unravelling mysteries in a detective’s investigation.

Understanding and solving this particular equation is important to unravelling pivotal clues in a case. It is the gateway to comprehending underlying principles governing physical phenomena and mathematical relationships, empowering us to predict outcomes and devise solutions to complex challenges.

Whether tasked with engineering marvels such as bridges, crafting exhilarating roller coasters, or simply seeking deeper insights into the fabric of reality, mastery of quadratic equations bestows a transformative ability—an intellectual superpower enabling individuals to unlock the enigmas of the universe. Thus, with an eagerness akin to superheroes unearthing hidden truths, let us embark on the journey to decode and solve this equation, embracing its profound implications for our understanding of the world.

Step-by-Step Guide to Solving “4x ^ 2 – 5x – 12 = 0”

Let’s delve into various methods to solve the quadratic equation “4x^2 – 5x – 12 = 0”, exploring different pathways to unlock its solutions:

Factoring Method:

Step 1: Identify two numbers whose product equals the product of the coefficient of x^2 (4) and the constant term (-12) while their sum equals the coefficient of x (-5).

Step 2: Rewrite the middle term (-5x) as the sum of two terms based on the identified numbers.

Step 3: Factor by grouping, finding the greatest common factor in each pair.

Step 4: Apply the zero-product property, setting each factor equal to zero and solving for x.

Quadratic Formula:

Step 1: Write down the quadratic formula: x = (-b ± √(b^2 – 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic equation.

Step 2: Identify the equation’s values of a, b, and c.

Step 3: Plug these values into the quadratic formula and simplify.

Step 4: Solve for x by calculating the two possible solutions using the ± symbol.

Completing the Square Method:

Step 1: Rewrite the equation in the form (x – h)^2 = k.

Step 2: Find the value of h by halving the coefficient of x and squaring the result.

Step 3: Complete the square by adding and subtracting h^2 inside the parentheses.

Step 4: Solve for x by taking the square root of both sides and isolating x.

Graphical Method:

Step 1: Plot the quadratic equation on a graph, with x on the horizontal axis and y on the vertical axis.

Step 2: Locate the x-intercepts, representing the solutions, where the graph intersects the x-axis.

Step 3: Read off the values of x from the graph to find the solutions.

By following these systematic approaches, you’ll unravel the solutions to “4x^2 – 5x – 12 = 0”, gaining a comprehensive understanding of quadratic equations. Let’s embark on this journey of exploration and problem-solving!

Common Problems Faced in Solving “4x ^ 2 – 5x – 12 = 0”

Navigating through quadratic equations like “4x^2 – 5x – 12 = 0” can present challenges. Here are some common hurdles you might encounter, along with strategies to overcome them:

Factoring Complexity:

Factoring quadratic equations involves identifying two numbers that multiply to the constant term and sum to the coefficient of x. However, this process can be intricate, particularly with equations featuring large coefficients or intricate terms.

Solution:

Take your time exploring different factor pairs, and consider employing methods like trial and error or the AC method to simplify the factoring process. If factoring becomes overly daunting, remember that alternative techniques, such as the quadratic formula or completing the square, remain viable options.

Handling Complex Roots:

Quadratic equations may yield real or complex roots contingent upon the discriminant within the quadratic formula. Dealing with complex roots may feel intimidating, especially if one is less familiar with imaginary numbers.

Solution:

Remain calm—complex roots are as valid as their counterparts and signify solutions within the complex number system. Should you feel uncertain about working with complex numbers, please take a moment to review fundamental concepts surrounding imaginary numbers and their properties. Consistent practice will reinforce comprehension.

Algebraic Mistakes:

Solving quadratic equations often involves numerous algebraic steps, such as simplifying expressions, combining like terms, and isolating variables. Missteps in these manipulations can lead to erroneous solutions.

Solution:

Exercise diligence and conduct thorough checks at each stage of the solving process. Pay meticulous attention to signs, correctly distribute terms, and employ parentheses where necessary to prevent errors. If any particular step raises uncertainty, take the time to revisit pertinent algebraic rules or seek clarification from a knowledgeable source.

Graphical Precision:

While the graphical method offers intuitive appeal, inaccuracies in plotting points or reading values off the graph may yield incorrect solutions. Moreover, quadratic equations with complex roots might not readily display identifiable intercepts on a graph.

Solution:

Exercise caution when plotting points on the graph,
utilizing graphing tools or software for enhanced accuracy. When equations yield complex roots, consider resorting to alternative methods like factoring or the quadratic formula for more precise solutions.

Acknowledging and strategizing around these hurdles’ll bolster your ability to confront quadratic equations like “4x^2 – 5x – 12 = 0” with assurance and accuracy. Remember, persistence and regular practice are integral to mastering mathematical challenges!

Frequently Asked Questions